Question

The exponential model Upper A equals 682.2 e Superscript 0.013 t describes the​ population, A, of a country in​ millions, t years after 2003. Use the model to determine when the population of the country will be 840 million.

Answer 1

The population will become 840 million in 2019.

Explanation:

Given:

The exponential model of population is given as:

`A=682.2e^(0.013t)`

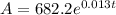
Here, 't' is in years measured since 2003.

This means for the year 2003, t = 0 and so on.

Now, in order to get the year when the population is 840 million, we need to plug in 840 for 'A' and solve for 't'. Therefore,

`840=682.2e^(0.013t)\\\\e^(0.013t)=(840)/(682.2)\\\\e^(0.013t)=1.2313`

Taking natural log on both sides, we get:

`0.013t=\ln(1.2313)\\\\0.013t=0.2081\\\\t=(0.2081)/(0.013)\\\\t=16\ years`

Therefore, 16 years after 2003, the population will be 840 million.

So, the year is equal to 2003 + 16 = 2019.

Hence, in the year 2019, the population will become 840 million.