Question

What is the magnitude of the electric field 3m away from the source if the electric potential is described by the function f(x)=2x+3, where x=2?

Answer 1

Hi there!

Recall the following relationship between the electric field and electrical potential:

`E = -(dV)/(dx)`

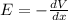
Begin by taking the derivative of the electric potential function using basic power and constant rules.

Remember:

`(dy)/(dx)x^n = nx^(n - 1)`

`(dy)/(dx)(C) = 0`

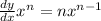
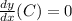
Differentiate the function, and take its negative.

`-(dV)/(dx) = -(2(1)(x^(1-1)) + 0 )\\\\-(dV)/(dx) = -2`

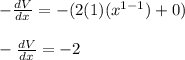
The magnitude is the absolute value, so:

`|(dV)/(dx) = |-2| = \boxed{2 (V)/(m)}`