Question

A car moves at speed v across a bridge made in the shape of a circular arc of radius r. (a) Find an expression for the normal force acting on the car when it is at the top of the arc. (b) At what minimum speed will the normal force become zero (causing the occupants of the car to seem weightless) if r 30.0 m

Answer 1

(a) FN = m (g -
`(v^(2) )/(r)`)

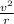(b) vmin = 17.146 m/s

Step-by-step explanation:

The radius of the arc is

r = 30m

The normal force acting on the car form the highest point is

FN = m (g -
`(v^(2) )/(r)`)

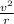If the normal force become 0 we have

m (g -
`(v^(2) )/(r)`) = 0

or

g -
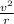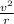`(v^(2) )/(r)` = 0

This way, when FN = 0, then v = vmin, so

g -
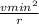`(vmin^(2) )/(r)` = 0

vmin =
`\sqrt[.]{g*r}` =
`\sqrt[.]{9.8 m/s^(2) * 30m } = 17.146 m/s`