Question

Consider the probability that exactly 20 out of 309 people will get the flu this winter. Choose the best description of the area under the normal curve that would be used to approximate binomial probability.

Answer 1

The distribution of proportion of people who will get the flu this winter is N (0.065, 0.014²).

Explanation:

Let X = number of people who will get flu this year.

The sample selected is of size, n = 309.

The number of people who will get flu in this sample is, x = 20.

Compute the sample proportion of people who will get flu as follows:

`p=(x)/(n)=(20)/(309)=0.065`

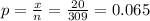
The random variable X follows a Binomial distribution with parameters n = 309 and p = 0.065.

The sample size is quite large, i.e. n = 309 > 30.

And the probability of success is low.

So the Normal approximation to Binomial can be used to approximate the distribution of sample proportion is:

1. np ≥ 10
2. n(1 - p) ≥ 10

Check whether the conditions are fulfilled or not as follows:

`np=309* 0.065=20.085>10\\n(1-p)=309* (1-0.065)=288.915>10`

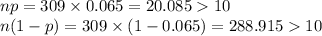
Hence, the conditions are fulfilled.

The sampling distribution of sample proportion is:

`p=N(p, (p(1-p))/(n))`

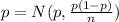
Compute the mean and variance as follows:

`Mean=p=0.065\\Variance=(p(1-p))/(n)=(0.065(1-0.065))/(309)=0.014^(2)`

Thus, the distribution of proportion of people who will get the flu this winter is N (0.065, 0.014²).