Answer:
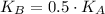
Step-by-step explanation:
Both carts are modelled by using the Principle of Energy Conservation and Work-Energy Theorem:
Cart A:
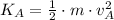
By assuming a constant acceleration, final velocity is equal to:
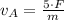
Then,
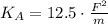
Cart B:
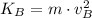
By assuming a constant acceleration, final velocity is equal to:

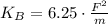
The kinetic energy of cart B is 0.5K.