Answer:
The diver will be 8 feet from the end of the board when he hits the water.
Explanation:
The diver hits the water when y = 0.
To find the distance, we have to find the values of x when y = 0.
Solving a quadratic equation:
Given a second order polynomial expressed by the following equation:
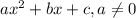 .
.
This polynomial has roots
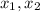 such that
such that
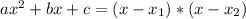 , given by the following formulas:
, given by the following formulas:

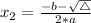

In this problem, we have that:

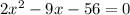
So
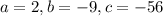
Then
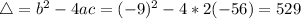


It is a horizontal distance, so the answer is a positive value.
The diver will be 8 feet from the end of the board when he hits the water.