Answer:
C. The converse of the Pythagorean theorem states that if the square of one side of a triangle is equal to the sum of the squares of the other two sides, then the triangle is a right triangle.
Explanation:
-That is, if in
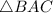 ,
,
 then ABC is said to be a right angle triangle.
then ABC is said to be a right angle triangle.
-
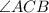 being the right angle measuring 90°.
being the right angle measuring 90°.
-Hence, C is the correct answer since the square of the hypoteneuse equals the sum of squares of the base and height.