Question

110 members of a sports club play at least one of d games football, basketball nd volley ball. if 20 play football and basketball, 15 play football nd volleyball only, 26 play basketball and volleyball. x play all the three games, 2x each play one game, how many play basketball all together?

​

Answer 1

`\boldsymbol{\mathbf{Answer}}`

`\boldsymbol{\mathbf{67\,members\, play\, basketball \, altogether}}`

`\boldsymbol{\mathbf{Step-by-step explanation}}`

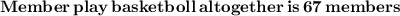
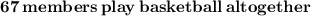
As Given ,

Members play football and basketball = 20

Members play football and vallyboll = 15

Members play basketball and volleyball = 26

Members play all games = x

Members play only one game = 2x

There are total number of members = 110

From above data we can write,

110 = 20 + 15 + 26 + x + 2x + 2x + 2x

110 = 61 + 7x

7x = 110 - 61

7x = 49

x = 7members

To find,

Member play basketboll altogether

= 20 + 26 + 2x + x

We know that x = 7,

= 20 + 26 + 14 + 7

= 67 members

`\boldsymbol{\mathbf{Member\, play\, basketboll\, altogether\, is\, 67\, members }}`