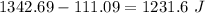Question

An 83.5 kg fullback moving east with a speed of 5.0 m/s is tackled by a 84.5 kg opponent running west at 2.66 m/s, and the collision is perfectly inelastic. (a) Calculate the velocity of the players just after the tackle. m/s (b) Calculate the decrease in kinetic energy during the collision. J

Answer 1

(a) 1.15 m/s

(b) 1231.60 J

Step-by-step explanation:

Given:

Mass of fullback (m) = 83.5 kg

Mass of opponent (M) = 84.5 kg

Initial velocity of fullback (u) = 5.0 m/s

Initial velocity of opponent (U) = -2.66 m/s

The collision is perfectly inelastic. So, both the fullback and opponent stick together after collision and travel with same velocity.

(a)

Let the final velocity be 'v'.

The total momentum before and after collision is conserved. Therefore,

Initial momentum = Final momentum

Initial momentum of fullback + opponent = Final momentum of fullback + opponent

`mu+MU=(m+M)v\\\\v=(mu+MU)/(m+M)`

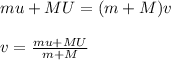
Plug in the given values and solve for 'v'. This gives,

`v=((83.5* 5.0)+(84.5* -2.66))/((83.5+84.5))\\\\v=(417.5-224.77)/(168)\\\\v=(192.73)/(168)=1.15\ m/s`

Therefore, the velocity of the players just after the tackle is 1.15 m/s.

(b)

The decrease in kinetic energy is given as:

`\Delta K=Initial\ KE\ of\ players-Final\ KE\ of\ players`

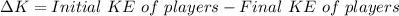
Initial kinetic of the players is given as:

`K_i=(1)/(2)mu^2+(1)/(2)mU^2\\\\K_i=(1)/(2)* 83.5* 5^2+(1)/(2)* 84.5* (-2.66)^2\\\\K_i=1043.75+298.94=1342.69\ J`

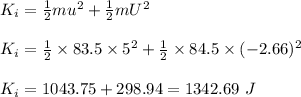
Final kinetic energy of the players is given as:

`K_f=(1)/(2)(m+M)v^2\\\\K_f=(1)/(2)(83.5+84.5)(1.15)^2\\\\K_f=111.09`

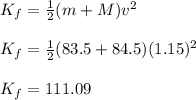
Therefore, the decrease in kinetic energy is given as:

Decrease =
`1342.69-111.09=1231.6\ J`