Answer:
Therefore n is divisible by 9 if and only if
 is also divisible by 9.
is also divisible by 9.
Explanation:
Given number is
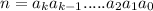
This means
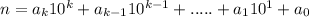
Here we need to prove
 is divisible by 9.
is divisible by 9.
We know that
10 ≡ 1 mod 9
It means if 10 divides by 9 the remainder = 1.
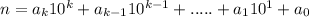
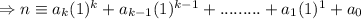 mod 9
mod 9
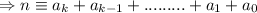 mod 9
mod 9
Therefore n is divisible by 9 if
 is also divisible by 9.
is also divisible by 9.
And conversely is also true.
Therefore n is divisible by 9 if and only if
 is also divisible by 9.
is also divisible by 9.