Question

A. Convert 5x^2 + 55x + 140 from standard to vertex form
b. Describe the transformation.
​

Answer 1

See below.

Explanation:

5x^2 + 55x + 140 ---> required

Coefficients a=5, b=55, c=140 yield

`h=(-h)/(2*a) =(-(55))/(2*5) =(-55)/(10) =-5.5`

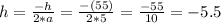
Thus, the x-coordinate of the vertex is
`\boxed{h = -5.5 }`

Plugging x=-5.5 into the given equation yields:

`k=5*(-5.5)^(2) +55*-5.5+140=-11.25`

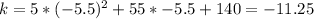
Thus, the y-coordinate of the vertex is
`\boxed{k = -11.25 }`

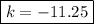
Altogether, the vertex of the given parabola is

`\boxed{ (h,k)=(-5.5,-11.25) }`

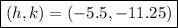
Plugging (h,k)=(−5.5,−11.25) into the vertex formula

`\boxed{ y=(x-h)^2+k }`

yields the vertex form of the parabola

`\boxed{ y=(x+5.5)^2-11.25 }`

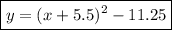
• To find the vertex of a parabola in standard form, first, convert it to the vertex form y=a(x−h)2+k y = a ( x − h ) 2 + k .

Answer 2

The transformation will be 5

Explanation: