The angles of the triangle are 39°, 51°, and 90°.
Explanation:
Step 1:
The sum of all the angles in any triangle is equal to 180°.
We have two of the three angles in terms of x while the third angle is not given directly. Since it is a right-angled triangle, one of the angles equals 90°.
So the angles of the triangle are
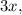
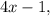 and
and
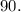
Step 2:
Now, we substitute all the angles to a sum of 180 to determine the value of x.
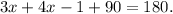
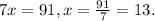
So x = 13°. The angles are

The angles of the triangle are 39°, 51°, and 90°.