Question:
(c) Boris also took a logic test. His z-score on that test was +0.93 . Does this change the answer to which test Boris performed better on? Explain your answer using z-scores.
Answer:
The answers to the questions are;
(a) Based on the z score, Boris perform better on his aptitude test.
(b) Based on the z score, Callie perform better on his knowledge test .
(c) For Boris since +0.93 =
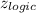 >
>
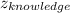 >
>
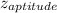
Yes as Boris now performed best on the logic test.
Explanation:
The z-score of a score is a measurement of the score withe respect to its distance from the mean as a factor of the standard deviation.
To solve the question, we note that we are required to find the z score as follows.
z score is given by
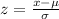
Where:
z = Standard score
x = Score
σ = Standard deviation
μ = Mean
(a) To find out which test did Boris performed better usin z score, we have
Boris scored a
57 on the knowledge test and
106 on the aptitude test
Therefore the z sore for the knowledge test is
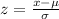
Here
x = 57
μ = 60
σ = 4.3
Therefore
 = -3/4.3 = -30/43 = -0.6977
= -3/4.3 = -30/43 = -0.6977
The z sore for Boris on the aptitude test is
Here
x = 106
μ = 110
σ = 7.1
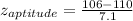 = -40/17 = -0.5634
= -40/17 = -0.5634
Based on the z score, Boris perform better on the aptitude test as his z score is higher (on the number line), --0.5634, compared to the z score on the knowledge test , -0.6977
(b) For Callie we have
Callie scored a
63 on the knowledge test and
114 on the aptitude test
Therefore the z sore for the knowledge test is
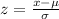
Here
x = 63
μ = 60
σ = 4.3
Therefore
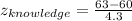 = 3/4.3 = 30/43 = 0.6977
= 3/4.3 = 30/43 = 0.6977
The z sore for Callie on the aptitude test is
Here
x = 114
μ = 110
σ = 7.1
 = 40/17 = 0.5634
= 40/17 = 0.5634
Based on the z score, Callie perform better on the knowledge test as his z score is higher (on the number line), 0.6977, compared to the z score on the aptitude test , 0.5634.
(c) If
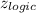 = +0.93 then sinc for Boris
= +0.93 then sinc for Boris
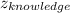 = -0.6977 and
= -0.6977 and
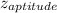 = - 0.5634 then
= - 0.5634 then
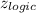 >
>
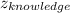 >
>
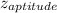
Therefore Boris now performed best on the logic test.