Question

A 25-kg iron block initially at 350oC is quenched in an insulated tank that contains 100 kg of water at 18oC. Assuming the water that vaporizes during the process condenses back in the tank, determine the total entropy change during this process.

Answer 1

The value of total entropy change during the process

`dS = 0.608 (KJ)/(K)`

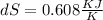
Step-by-step explanation:

mass of iron
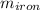`m_(iron)` = 25 kg

Initial temperature of iron
`T_(1)` = 350°c = 623 K

Mass of water
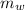`m_(w)` = 100 kg

Initial temperature of water
`T_(2)` = 180°c = 453 K

When iron block is quenched inside the water the final temperature of both iron & water becomes equal. this is =
`T_(f)`

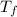
Thus heat lost by the iron block = heat gain by the water

⇒
`m_(iron)`
`C_(iron)` (
`T_(1)` -
`T_(f)` ) =
`m_(w)`
`C_(w)` (
`T_(f)` -
`T_(2)` )

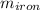
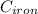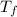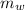
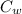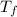⇒ 25 × 0.448 × (
`T_(1)` -
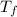`T_(f)` ) = 100 × 4.2 × (
`T_(f)` -
`T_(2)` )

⇒
`( T_(1) - T_(f) ) = 37.5 ( T_(f) - T_(2) )`

⇒
`( 623 - T_(f) ) = 37.5 ( T_(f) - 453 )`

⇒
`( 623 - T_(f) ) = 37.5 T_(f) - 16987.5`

⇒
`38.5 T_(f) = 17610.5`

⇒
`T_(f) = 457.41 K`

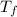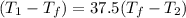
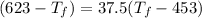
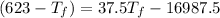
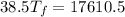
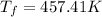
This is the final temperature after quenching.

The total entropy change is given by,

`dS = m_(iron)\ C_(iron) \ ln (T_(f) )/(T_(1) ) + m_(w)\ C_(w) \ ln (T_(f) )/(T_(2) )`

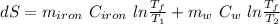
Put all the values in above formula,

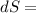`dS =` 25 × 0.448 ×
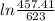`ln (457.41)/(623)` + 100 × 4.2 ×
`ln \frac {457.41}{453}`

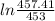
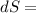`dS =` - 3.46 + 4.06

`dS = 0.608 (KJ)/(K)`

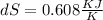
This is the value of total entropy change.