Question

A group of students bakes 100 cookies to sell at the school bake sale. The students want to ensure that the price of each cookie offsets the cost of the ingredients. If all the cookies are sold for $0.10 each, the net result will be a loss of $4. If all the cookies are sold for $0.50 each. The students will make a $36 profit. First, write the linear function p(x) that represents the net profit from selling all the cookies, where x is the price of each cookie. Then, determine how much profit the students will make if they sell the coolies for $0.60 each. Explain. Tell how your answer is reasonable.

Answer 1

(a)
`p=100x-14`

(b)
`p=\$46`

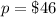
Explanation:

Linear Modeling

It consists of finding an equation of a line that fits the conditions of a certain situation in real life. We'll use a linear model for the cookies of the students.

(a) We know that we have a total of n=100 cookies. If sold for $0.10 each, they lose $4. We have an initial condition (x,p) = (0.10,-4), where x is the price of each cookie and p(x) is the net profit from selling all the cookies. The second conditions are that when then the price is $0.50 each, there is a positive profit of $36, which is a second point (0.5,36). That is enough to build the linear function, that can be found by

`\displaystyle p-p_1=(p_2-p_1)/(x_2-x_1)(x-x_1)`

`\displaystyle p+4=(36+4)/(0.5-0.1)(x-0.1)`

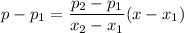
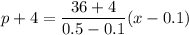
Reducing

`p=100x-14`

(b) If the students sell the cookies for x=0.60 each, the profit will be

`p=100(0.6)-14=46`

`p=\$46`

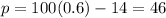
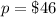
It's a reasonable answer because we have found that increasing the price, the profit will increase also. The model doesn't have any restriction for the price