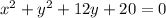Answer:
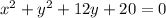
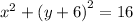
Explanation:
The equation of a circle with center (a,b) and radius r, is given by:
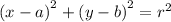
The given circle has centre (0,-6) and radius r=4.
We substitute these values into the formula to get:
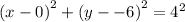
This implies that:
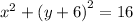
When we expand, we get:
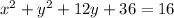
The general form is :