Question

to arrive at 2000 liters of 60% saline solution the attendant has to mix a 90% and a 40% saline solution. How many liters of each should be used?

Answer 1

800 liters of 90% saline solution and 1200 liters of 40% saline solution should be used.

Explanation:

Given:

At 2000 liters of 60% saline solution the attendant has to mix a 90% and a 40% saline solution.

Now, to find the number of liters of saline solution each should be used.

Let the liters of 90% saline solution mix be
`x.`

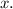
And let the liters of 40% saline solution mix be
`y.`

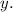
So, the total number of liters:

`x+y=2000.`

`y=2000-x\ \ \ ....(1)`

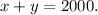
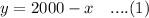
Now, the total percentage of saline solution:

`90\%\ of\ x+40\%\ of\ y=60\%\ of\ 2000`

`(90)/(100)* x+(40)/(100)* y=(60)/(100)* 2000`

`0.9x+0.4y=1200`

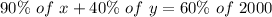
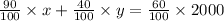
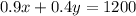
Substituting the value of
`y` from equation (1) we get:

`0.9x+0.4(2000-x)=1200`

`0.9x+800-0.4x=1200`

`0.5x+800=1200`

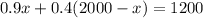
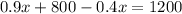
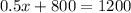
Subtracting both sides by 800 we get:

`0.5x=400`

Dividing both sides by 0.5 we get:

`x=800.`

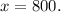
The liters of 90% saline solution mix = 800.

Now, substituting the value of
`x` in equation (1) to get the liters of 40% saline solution:

`y=2000-x`

`y=2000-800`

`y=1200.`

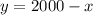
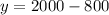
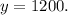
Thus, the liters of 40% saline solution = 1200.

Therefore, 800 liters of 90% saline solution and 1200 liters of 40% saline solution should be used.