Answer:
The conductivity of Nichrome is
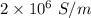 .
.
Step-by-step explanation:
Given:
Potential difference (V) = 2.0 V
Current flowing (I) = 4.0 A
Length of wire (L) = 1.0 m
Area of cross section of wire (A) = 1.0 mm² = 1 × 10⁻⁶ m² [1 mm² = 10⁻⁶ m²]
We know, from Ohm's law, that the ratio of voltage and current is always a constant and equal to the resistance of the resistor. Therefore, the resistance of the nichrome wire is given as:

Now, resistance of the nichrome wire in terms of its resistivity, length and area of cross section is given as:
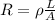
Where,
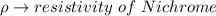
Now, plug in all the values given and solve for
 . This gives,
. This gives,
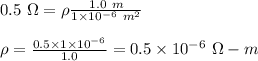
Now, conductivity of a material is the reciprocal of its resistivity. Therefore, the conductivity of Nichrome is given as:
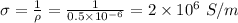
Conductivity is measured in Siemens per meter (S/m)
Therefore, the conductivity of Nichrome is
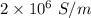 .
.