Question

Gina's literacy bucket weighs 6 pounds. Her novel weighs 1 4/6 pounds, and her chrome book weighs 2 1/6 how much would her bucket weigh if she took out the two items.

Answer 1

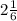`2(1)/(6)` pounds.

Explanation:

We have been given that Gina's literacy bucket weighs 6 pounds. Her novel weighs 1 4/6 pounds, and her chrome book weighs 2 1/6.

To find weight of bucket after taking out the two items, we will subtract weight of each item from 6 pounds as:

`\text{Weight of bucket}=6-1(4)/(6)-2(1)/(6)`

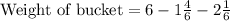
Let us convert mixed fractions into improper fractions as:

`1(4)/(6)=(6\cdot 1+4)/(6)=(10)/(6)\\\\2(1)/(6)=(6\cdot 2+1)/(6)=(12+1)/(6)=(13)/(6)`

`\text{Weight of bucket}=6-(10)/(6)-(13)/(6)`

`\text{Weight of bucket}=(6\cdot 6)/(6)-(10)/(6)-(13)/(6)`

`\text{Weight of bucket}=(36)/(6)-(10)/(6)-(13)/(6)`

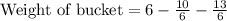
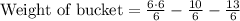
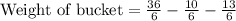
Combine numerators:

`\text{Weight of bucket}=(36-10-13)/(6)`

`\text{Weight of bucket}=(13)/(6)`

`\text{Weight of bucket}=2(1)/(6)`

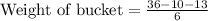
Therefore, the weight of the bucket is
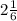`2(1)/(6)` pounds.