Question

Two-variable inequalities word problems Go to lesson page Problem Michelle has a maximum of 4500 45004500 milliliters ( ml ) (ml)left parenthesis, start text, m, l, end text, right parenthesis of water for her plants today. Each basil plant requires 350 ml 350 ml350, start text, space, m, l, end text of water, and each fennel plant requires 525 ml 525 ml525, start text, space, m, l, end text of water.

Answer 1

<img src="
`image`

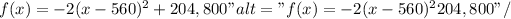
Explanation:

Let

f(x) ----> the profit earned

x ---> is the number of orders fulfilled

we know that

The equation of a quadratic equation in vertex form is equal to

where

a is the leading coefficient of the quadratic equation

(h,k) is the vertex

Remember that

The x-intercepts or roots are the values of x when the value of the function is equal to zero

In this problem

The x-intercepts are

x=240 and x=880

The x-coordinate of the vertex (h) is the midpoint of the roots

so

The y-coordinate of the vertex (k) is the maximum profit earned

----> is given

so

The vertex is the point (560,204,800)

substitute in the quadratic equation

Find the value of a

we have the ordered pairs (240,0) and (880,0) (the x-intercepts)

take the point (240,0) and substitute the value of x and the value of y in the quadratic equation

solve for a

therefore

The function that models the profits of the company is given by

----> equation in verte

Answer 2

Explanation:

Below is an attachment containing the solution