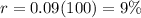Question

William deposits S2 170 into an interest-bearing account that is compounded continuously He decides to not deposit or withdraw

any money after the initial deposit. The following function represents the balance of the account which is compounded continuously
at a rate ofr after years
A(t) = $2,170 ert)
If the balance of the account is $2.843 after 3 years, then what is the approximate interest rate of the account?

Answer 1

The approximate interest rate of the account is r=0.09 or r=9%

Explanation:

we know that

The formula to calculate continuously compounded interest is equal to

`A=P(e)^(rt)`

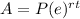
where

A is the Final Investment Value

P is the Principal amount of money to be invested

r is the rate of interest in decimal

t is Number of Time Periods

e is the mathematical constant number

we have

`t=3\ years\\ P=\$2,170\\A=\$2,843`

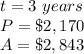
substitute in the formula above

`2,843=2,170(e)^(3r)`

Solve for r

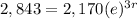
`(2,843/2,170)=(e)^(3r)`

Apply ln both sides

`ln(2,843/2,170)=ln[(e)^(3r)]`

Applying property of exponents

`ln(2,843/2,170)=(3r)ln[(e)`

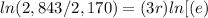
Remember that

`ln(e)=1`

`ln(2,843/2,170)=(3r)`

`r=ln(2,843/2,170)/(3)`

`r=0.09`

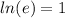
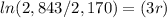
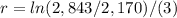
Convert to percentage

`r=0.09(100)=9\%`