Answer:
x² + y² - 12x - 16y = 280
Explanation:
The diameter of a circle has endpoints A(-8,-5) and B(4,11)
The equation of a circle is given by: (x - a)² + (y - b)² = r² , Where x and y are points on the circle and (a, b) is the center of the circle. r is the radius.
To find the center of the circle:
Center = (
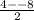 ,
,
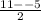 ) = (6, 8)
) = (6, 8)
The length of the diameter is the square root of change in y squared plus change in x squared.
The length of the diameter squared = (11 - -5)² + (4 - -8)² = 400
The diameter is
![\sqrt[]{400}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/jhnf5v98k2fkyl7lldzq6tcwz5ol0zogxy.png) = 20 units
= 20 units
Taking another point (x,y) on the circle,
The equation of the circle will be:
(x - 6)² + (y - 8)² = 20²
Simplifying this gives;
x² - 12x + 36 + y² - 16y + 84 = 400
x² + y² - 12x - 16y = 400 - 84 - 36
x² + y² - 12x - 16y = 280