Good morning ☕️
Answer:
the negative solution is -2
Explanation:
First ,we need to model the situation by expressing it as an equation
so, let x represent the uncknown negative number :
We get
x² - 10 = 3x
then
x² - 3x - 10 = 0
now using the quadratic formula:
Let Δ be the discriminant
Δ = b² - 4ac = (-3)² - 4(1)(-10) = 9 + 40 = 49 → √∆ = 7
Therefore
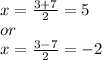
_______________________________________
:)