Answer:
(a) We reject the null hypothesis that usual load is 13 credits.
(b) Probability that student at this college takes 16 or more credits = 0.10935
Explanation:
We are given that the histogram below shows the distribution of the number of credits taken by these students;
Min Q1 Median Mean SD Q3 Max
8 13 14 13.65 1.91 15 18
Also, the counselor decides to randomly sample 100 students by using the registrar's database of students, i.e., n = 100.
So, Null Hypothesis,
 :
:
 = 13 {means that the usual load is 13 credits}
= 13 {means that the usual load is 13 credits}
Alternate Hypothesis,
 :
:
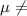 13 {means that the usual load is not 13 credits}
13 {means that the usual load is not 13 credits}
The test statistics we will use here is ;
T.S. =
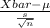 ~
~

where, Xbar = sample mean = 13.65
s = sample standard deviation = 1.91
n = sample size = 100
So, test statistics =
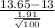 ~
~
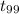
= 3.403
Now, since significance level is not given to us so we assume it to be 5%.
At 5% significance level, the t tables gives critical value of 1.987 at 99 degree of freedom. Since our test statistics is more than the critical value which means our test statistics will lie in the rejection region, so we have sufficient evidence to reject null hypothesis.
Therefore, we conclude that the usual load is not 13 credits.
(b) Let X = credits of students
The z score probability distribution is given by;
Z =
 ~ N(0,1)
~ N(0,1)
So, probability that student at this college takes 16 or more credits = P(X
 16)
16)
P(X
 16) = P(
16) = P(


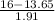 ) = P(Z
) = P(Z
 1.23) = 1 - P(Z < 1.23)
1.23) = 1 - P(Z < 1.23)
= 1 - 0.89065 = 0.10935 or 11%
Therefore, probability that student at this college takes 16 or more credits is 0.10935.