Question

Suppose ACT Reading scores are normally distributed with a mean of 21.4 and a standard deviation of 6.2. A university plans to award scholarships to students whose scores are in the top 8%. What is the minimum score required for the scholarship? Round your answer to the nearest tenth, if necessary.

Answer 1

im sorry

Explanation:

Answer 2

30.1

Explanation:

Mean score (μ) = 21.4

Standard deviation (σ) = 6.2

If only the top 8% of scores will get scholarships, students whose scores are at the 92nd percentile or above qualify for a scholarship. In a normal distribution, the 92nd percentile has a corresponding z-score of z = 1.405.

The minimum score, X, required for a scholarship is given by:

`z=(X-\mu)/(\sigma) \\1.405=(X-21.4)/(6.2)\\ X=30.1`

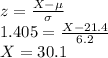
The minimum score required for the scholarship is 30.1.