Answer:
The value of the test statistic is 1.667
Explanation:
We are given that the sales of a grocery store had an average of $8,000 per day. The store introduced several advertising campaigns in order to increase sales. For this a random sample of 64 days of sales was selected. It was found that the average was $8,250 per day. From past information, it is known that the standard deviation of the population is $1,200.
We have to determine whether or not the advertising campaigns have been effective in increasing sales.
Let, Null Hypothesis,
 :
:
 = $8,000 {means that the advertising campaigns have not been effective in increasing sales}
= $8,000 {means that the advertising campaigns have not been effective in increasing sales}
Alternate Hypothesis,
 :
:
 > $8,000 {means that the advertising campaigns have been effective in increasing sales}
> $8,000 {means that the advertising campaigns have been effective in increasing sales}
The test statistics that will be used here is One sample z-test statistics;
T.S. =
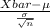 ~ N(0,1)
~ N(0,1)
where, Xbar = sample mean = $8,250
 = population standard deviation = $1,200
= population standard deviation = $1,200
n = sample size = 64
So, test statistics =

= 1.667
Therefore, the value of test statistics is 1.667 .