Answer:
Option D) significantly different from 24
Explanation:
We are given the following in the question:
Population mean, μ = 24
Sample mean,
 = 25
= 25
Sample size, n = 16
Alpha, α = 0.05
Population standard deviation, σ = 2
First, we design the null and the alternate hypothesis

We use Two-tailed z test to perform this hypothesis.
Formula:
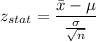
Putting all the values, we have

Now,
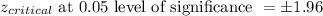
Since,
The calculated z statistic does not lie in the acceptance region, we fail to accept the null hypothesis and reject it. We accept the alternate hypothesis.
Thus, it can be concluded that the mean age is
Option D) significantly different from 24