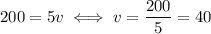Question

Jill drove the 200 miles to Cincinnati at an average speed of 10 miles per hour faster than her usual average speed. If she completed the trip in 1 hour less than usual, what is her usual driving speed, in miles per hour

Answer 1

Let
`v` be the usual speed, and
`t` be the usual time.

Following the equation
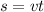`s=vt`, where s is the space, v is the velocity and t is time, we know that usually we have

`200=vt`

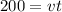
But this time we were 10mph faster and it took one hour less, so we have

`200=(v+10)(t-1)`

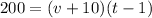
Since both right hand sides equal 200, they must equal each other:

`vt=(v+10)(t-1)=vt+10t-v-10 \iff 10t-v-10=0 \iff v=10t-10`

Plug this value in the first equation and we have

`200=vt=(10t-10)t \iff 10t^2-10t-200=0`

This equation has solutions
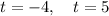`t=-4,\quad t=5`. We can only accept positive solutions, so we have t=5. We finally deduce, again from the first equation,

`200=5v \iff v=(200)/(5)=40`