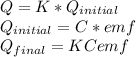Question

A resistor with resistance R and an air-gap capacitor of capacitance C are connected in series to a battery (whose strength is "emf").

(a) What is the final charge on the positive plate of the capacitor? (Use the following as necessary: C, emf.)

Q =___________
(b) After fully charging the capacitor (so there is no current), a sheet of plastic whose dielectric constant is K is inserted into the capacitor and fills the gap. Explain why a current starts running in the circuit. You can base your explanation either on electric field or on electric potential, whichever you prefer.
This answer has not been graded yet.
(c) What is the initial current through the resistor just after inserting the sheet of plastic? (Use the following as necessary: R, K, emf. Note that the K is an upper-casek.)
I =________
(d) What is the final charge on the positive plate of the capacitor after inserting the plastic? (Use the following as necessary: C, K, emf. Note that the K is an upper-case k.)
Qnew =________

Answer 1

a) Q = C*emf

b) Reduction in electric field strength and electric potential

c) Initial current through the resistor = emf/R

d) The final charge = K*C*emf

Step-by-step explanation:

a) The resistors and capacitors are connected in series with the battery

Using Kirchoff's voltage law, sum of all voltages in the circuit is zero

Let
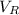`V_(R)` = Voltage dropped across the Resistor

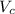`V_(c)` = Voltage dropped across the capacitor

Applying KVL;

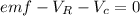`emf - V_(R) - V_(c) = 0\\`.........................(1)

Since the connection is in series, the same current flow through the circuit

`V_(R) = IR\\Q = CV_(c) \\V_(c) = Q/C`

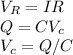
Putting
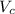`V_(c)` and
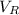`V_(R)` into equation (1)

`emf - IR - Q/C = 0`

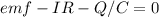
At the final charge, the capacitor in fully charged, and current drops to zero due to equilibrium

`I = 0A\\emf = Q/C\\Q = C* emf`

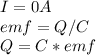
b) Current starts running through the plate because as the sheet of plastic is inserted between the plates both the electric field intensity and the electric potential reduces. The charge also reduces, then current flows

c) The current through the resistor is the current through the entire circuit ( series connection)

`I = I_(o) \exp((-t)/(RC) )\\At time the initial time, t\\t = 0\\ I_(o) = (emf)/(R) \\`

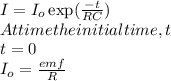
Putting the values of t and I₀ into the formula for I written above

`I = (emf)/(R) \exp(0)\\I = (emf)/(R)`

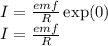
d) NB: The initial charge on the capacitor = C * emf

The final charge will be:

`Q = K* Q_(initial) \\Q_(initial) = C *emf\\Q_(final) = KCemf`