Question

The density of mobile electrons in copper metal is 8.4 1028 m-3. Suppose that i = 4.6 1018 electrons/s are drifting through a copper wire. (This is a typical value for a simple circuit.) The diameter of the wire is 1.2 mm. In this case, about how many minutes would it take for a single electron in the electron sea to drift from one end to the other end of a wire 31 cm long?

Answer 1

The time is 106.7 minute.

Step-by-step explanation:

Given that,

Density
`= 8.4*10^(28)\ m^3`

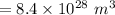
Current
`i = 4.6*10^(18)\ electron/s`

Diameter of wire = 1.2 mm

Length = 31 cm

We need to calculate the drift velocity

Using formula of drift velocity

`v_(d)=(I)/(neA)`

`v_(d)=(Ne)/(tne*\pi r^2)`

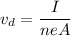
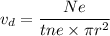
Put the value into the formula

`v_(d)=(4.6*10^(18))/(8.4*10^(28)*\pi*(0.6*10^(-3))^2)`

`v_(d)=4.842*10^(-5)\ m/s`

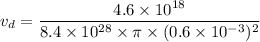
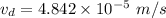
We need to calculate the time

Using formula for time

`v_(d)=(l)/(t)`

`t=(l)/(v_(d))`

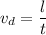
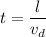
Where, l = length

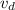`v_(d)` = drift velocity

Put the value into the formula

`t=(31*10^(-2))/(4.842*10^(-5))`

`t=6402.31\ sec`

`t=106.7\ minute`

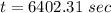
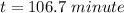
Hence, The time is 106.7 minute.