Question

I need help asap

Which equation represents a circle with diameter endpoints at (−3,−2) and (1,−4)?


A

(x−1)2+(y−3)2=20


B

(x+1)2+(y+3)2=5


C

(x+1)2+(y+3)2=20


D

(x−1)2+(y−3)2=5

Answer 1

Option C:
`(x+1)^2+(y+3)^2=20` is the equation of the circle.

Step-by-step explanation:

Given that the endpoints of the circle are at (-3,-2) and (1,-4)

The equation of the circle can be determined using the formula,

`(x-a)^(2)+(y-b)^(2)=r^(2)`

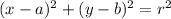
where
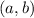`(a, b)` are the coordinates of the center and r is the radius.

Center:

The center of the circle can be determined using the midpoint formula,

`Center=((x_1+x_2)/(2), (y_1+y_2)/(2) )`

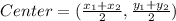
Substituting the coordinates (-3,-2) and (1,-4) in the above formula, we get,

`Center=((-3+1)/(2), (-2-4)/(2) )`

`Center=((-2)/(2), (-6)/(2) )`

`Center=(-1,-3 )`

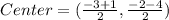
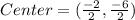
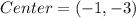
Thus, the center of the circle is at (-1,-3)

Radius:

The radius of the circle can be determined using the distance formula,

`r=\sqrt{\left(x_(2)-x_(1)\right)^(2)+\left(y_(2)-y_(1)\right)^(2)}`

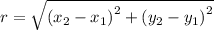
Substituting the coordinates (-3,-2) and (1,-4) in the above formula, we get,

`r=\sqrt{\left(1+3\right)^(2)+\left(-4+2\right)^(2)}`

`r=\sqrt{\left(4\right)^(2)+\left(-2\right)^(2)}`

`r=√(\left16+\left4)`

`r=√(20)`

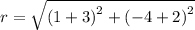
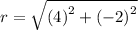
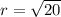
Thus, the radius of the circle is
`√(20)`

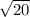
Equation of the circle:

Substituting the center and the radius of the circle in the equation
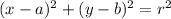`(x-a)^(2)+(y-b)^(2)=r^(2)`, we get,

`(x+1)^2+(y+3)^2=(√(20) )^2`

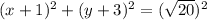
Simplifying, we get,

`(x+1)^2+(y+3)^2=20`

Therefore, the equation of the circle is
`(x+1)^2+(y+3)^2=20`

Hence, Option C is the correct answer.