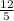Question

If secθ = 13\5 and 270° < θ < 360°, then tanθ = _____. -12\5 -5\12 5\12 12\5

Answer 1

tanΘ = -
`(12)/(5)`

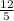
Explanation:

Using the trigonometric identity

tan²Θ + 1 = sec²Θ, thus

tan²Θ + 1 = (
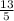`(13)/(5)` )² =
`(169)/(25)` ( subtract 1 from both sides )

tan²Θ =
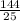`(144)/(25)` ( take the square root of both sides )

tanΘ = ±
`\sqrt{(144)/(25) }`

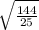
Since 270 < Θ < 360 , that is the fourth quadrant where tan Θ < 0, thus

tanΘ = -
`(12)/(5)`