A mass weighing 32 pounds stretches a spring 2 feet.
(a) Determine the amplitude and period of motion if the mass is initially released from a point 1 foot above the equilibrium position with an upward velocity of 6 ft/s.
(b) How many complete cycles will the mass have completed at the end of 4 seconds?
Answer:
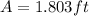
Period =
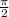 seconds
seconds
8 cycles
Step-by-step explanation:
A mass weighing 32 pounds stretches a spring 2 feet;
it implies that the mass (m) =
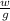
m=
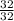
= 1 slug
Also from Hooke's Law
2 k = 32
k =
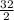
k = 16 lb/ft
Using the function:
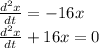
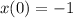 (because of the initial position being above the equilibrium position)
(because of the initial position being above the equilibrium position)
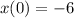 ( as a result of upward velocity)
( as a result of upward velocity)
NOW, we have:

However;
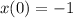 means
means

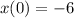 also implies that:
also implies that:

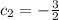
Hence,
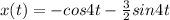
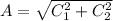
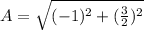
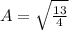
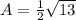
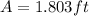
Period can be calculated as follows:
=
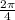
=
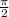 seconds
seconds
How many complete cycles will the mass have completed at the end of 4 seconds?
At the end of 4 seconds, we have:
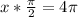
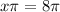
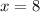 cycles
cycles