Question

A van starts off 152 miles directly north from the city of Springfield. It travels due east at a speed of 25 miles per hour. After travelling 91 miles, how fast is the distance between the van and Springfield changing

Answer 1

The speed at which the distance between the van and Springfield is changing is 25 miles per hour.

Step-by-step explanation:

To find the speed at which the distance between the van and Springfield is changing, we can use the concept of rate of change. Let's consider the van's journey as a right-angled triangle, with the straight distance from the van to Springfield as the hypotenuse. The van moved east and traveled 91 miles, which is one of the legs of the triangle. The remaining distance, the other leg, can be found using the Pythagorean theorem: √((152)² - (91)²) ≈ 114 miles.

Now, we need to find the rate at which the hypotenuse is changing. This can be done by differentiating the Pythagorean theorem equation for time. The rate at which the hypotenuse is changing, or the speed at which the distance between the van and Springfield is changing, can be found using the chain rule of differentiation.

Since the van is traveling east at a constant speed of 25 miles per hour, the distance between the van and Springfield is changing at a constant rate of 25 miles per hour.

Answer 2

12.84 miles per hour

Step-by-step explanation:

Given:

Vertical distance of starting point of van from Springfield (d) = 152 miles

Speed in east direction (s) = 25 mph

Distance traveled in east direction (e) = 91 miles

Let the direct distance from Springfield of the van be 'x' at any time 't'.

Now, from the question, it is clear that, the vertical distance of van is fixed at 152 miles and only the horizontal distance is changing with time.

Now, consider a right angled triangle SNE representing the given situation.

Point S represents Springfield, N represents the starting point of van and E represents the position of van at any time 't'.

SN = d = 152 miles (fixed)

Now, using the pythagorean theorem, we have:

`SE^2=SN^2+NE^2\\\\x^2=d^2+e^2\\\\x^2=(152)^2+e^2----(1)`

Now, differentiating both sides with respect to time 't', we get:

`2x(dx)/(dt)=0+2e(de)/(dt)\\\\(dx)/(dt)=(e)/(x)(de)/(dt)`

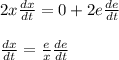
Now, we are given speed as 25 mph. So,
`(de)/(dt)=25\ mph`

Also, when
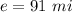`e=91\ mi`, we can find 'x' using equation (1). This gives,

`x^2=23104+(91)^2\\\\x=√(31385)=177.16\ mi`

Now, plug in the values of 'e' and 'x' and solve for
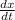`(dx)/(dt)`. This gives,

`(dx)/(dt)=(91)/(177.16)* 25\\\\(dx)/(dt)=12.84\ mph`

Therefore, the distance between the van and Springfield is changing at a rate of 12.84 miles per hour