Question

A 5.93 5.93 kg ball is attached to the top of a vertical pole with a 2.03 2.03 m length of massless string. The ball is struck, causing it to revolve around the pole at a speed of 4.25 4.25 m/s in a horizontal circle with the string remaining taut. Calculate the angle, between 0° and 90°, that the string makes with the pole. Take g = 9.81 g=9.81 m/s2.

Answer 1

42.2°

Step-by-step explanation:

Given:

Mass of the ball (m) = 5.93 kg

Length of string (L) = 2.03 m

Speed of ball (v) = 4.25 m/s

Acceleration due to gravity (g) = 9.81 m/s²

Let the angle with the vertical pole be 'x'. Let 'T' be the tension in the string.

Draw a free body diagram of the ball labeling all the forces on it.

From the diagram, the net force in the vertical force must be zero as there is no motion in the vertical direction.

So,
`T\cos x =mg------(1)`

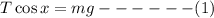
Now, the horizontal component of tension provides the necessary centripetal force for the ball to move in a circle. So,

`T\sin x=(mv^2)/(L)------- (2)`

Now, divide equation (2) by equation (1). This gives,

`(T\sin x)/(T\cos x)=(mv^2)/(L)/ mg\\\\\tan x=(mv^2)/(L)* (1)/(mg)\\\\\tan x=(v^2)/(Lg)`

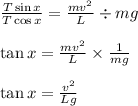
Now, plug in the given values and solve for 'x'. This gives,

`\tan x=((4.25)^2)/(2.03* 9.81)\\\\\tan x=0.907\\\\x=\tan^(-1)(0.907)=42.2\°`

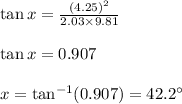
Therefore, the angle made by the string with the pole is 42.2°.