Question

g A particle starts moving from the origin of the coordinate system with the initial velocity v(0)=<0,0,2> and acceleration at time t given by a(t)=<1,2,0> find the moment of time t=T when the particle hits the plane 2x+y-z=4

Answer 1

2s

Step-by-step explanation:

The position function of the motion can be expressed as:

`s = s_0 + v_0t + at^2/2`

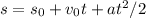
where
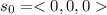`s_0 = <0,0,0>` is the origin where the particle starts off,
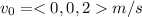`v_0 = <0,0,2> m/s` and a = <1,2,0> m/s2. In the 3-coordinate system it can be written as

`s = <0 + 0t+ t^2/2, 0 + 0t + 2t^2/2, 0 + 2t + 0t^2/2> = <t^2/2, t^2, 2t>`

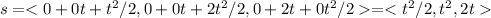
For the particle to hit the 2x+y-z=4 plane then its coordinates must meet that criteria

`2t^2/2 +t^2-2t = 4`

`2t^2 - 2t -4 = 0`

`t^2 - t - 2 = 0`

`t= (-b \pm √(b^2 - 4ac))/(2a)`

`t= (1\pm √((-1)^2 - 4*(1)*(-2)))/(2*(1))`

`t= (1\pm3)/(2)`

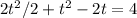
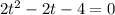
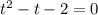
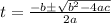
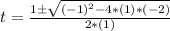
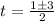
t = 2 or t = -1

Since t can only be positive we will pick t = 2s