Question

Suppose X is the time it takes a randomly chosen clerical worker in an office to type and send a standard letter of recommendation. Suppose X has a normal distribution, and assume the mean is 10.5 minutes and the standard deviation 3 minutes. You take a random sample of 50 clerical workers and measure their times. What is the chance that their average time is less than 9.5 minutes?

Answer 1

The chance that their average time is less than 9.5 minutes is 0.0094

Explanation:

X is the time it takes a randomly chosen clerical worker in an office to type and send a standard letter of recommendation

We are given that X has a normal distribution

Mean
`\mu` = 10.5 minutes

Standard deviation
`\sigma`= 3 minutes

n = 50

We are supposed to find the chance that their average time is less than 9.5 minutes i.e. P(X<9.5 minutes)

Formula :
`Z = (x-\mu)/((\sigma)/(√(n)))`

`Z = (9.5-10.5)/((3)/(√(50)))`

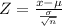
Z = −2.35

Refer the z table for p value

p value=0.0094

So, P(X<9.5)=P(Z<−2.35)=0.0094

Hence the chance that their average time is less than 9.5 minutes is 0.0094.