Question

The table below represents a linear function f(x) and the equation represents a function g(x):

x f(x)
−1 −12
0 −6
1 0
g(x)

g(x) = 2x + 6


Part A: Write a sentence to compare the slope of the two functions and show the steps you used to determine the slope of f(x) and g(x).
Part B: Which function has a greater y-intercept? Justify your answer.

Answer 1

Part A) The slope of the function f(x) is greater than the slope of the function g(x) (see the explanation)

Part B) Function g(x) has a greater y-intercept than the function f(x) (see the explanation)

Explanation:

Part A)

step 1

Find the slope of function f(x)

Find the slope m

The formula to calculate the slope between two points is equal to

`m=(y2-y1)/(x2-x1)`

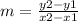
take the points

(0,-6) and (1,0)

substitute in the formula

`m=(0+6)/(1-0)`

`m=(6)/(1)=6`

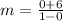
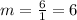
step 2

Find the slope of function g(x)

we have

`g(x)=2x+6`

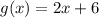
This is the equation of the line in slope intercept form

`y=mx+b`

where

m is the slope

b is the intercept

In this problem we have

`m=2\\b=6`

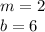
step 3

Compare the slopes

The slope of f(x) is m=6

The slope of g(x) is m=2

therefore

The slope of the function f(x) is greater than the slope of the function g(x)

Part B) Which function has a greater y-intercept?

we know that

The y-intercept is the value of y when the value of x is equal to zero

step 1

Determine the y-intercept of the function f(x)

Looking at the data in the table

For x=0, f(x)=-6

therefore

The y-intercept of f(x) is -6

step 2

Determine the y-intercept of the function g(x)

we have

`g(x)=2x+6`

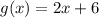
The y-intercept of the function g(x) is 6 (see part A)

Compare the y-intercept

6 > -6

therefore

Function g(x) has a greater y-intercept than the function f(x)