Question

Find the width of a rectangle with perimeter 92 and length 19

Answer 1

Explanation:

perimeter = l + b + l + b or 2(l + b)

92 = 19 + 19 + 2b

92 = 38 + 2b

2b = 92 - 38

2b = 54

b = 54 / 2

b = 27

breadth or width of the triangle = 27

length = 19

Answer 2

Width of the rectangle is 27

Explanation:

Given:

`Perimeter= 92 units\\\\Length= 19 units`

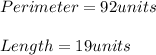
Perimeter of the rectangle =
`2(Length+Width)`

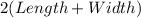
Putting the values:

`92=2(19+Width)`

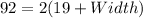
Dividing by '2' both the sides:

`92/2=19+width`

`46=19+Width`

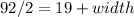
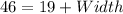
Subtracting '19' both the sides:

`46-19=Width`

`Width=27 units`

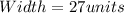
The width of the rectangle is 27 units with Length=19 units and Perimeter= 92 units