Answer:
DE = 3 units
Explanation:
The image is attached.
There are 2 secant lines in the circle. We can use secant theorem to solve this easily.
It states that "if 2 secants are drawn to a circle from an outside point, then product of 1 secant and its "outside" part is equal to product of other secant and its "outside" part.
From the figure, we can say:
AX * BX = EX * DX
We let the length to find , DE, be "x".
Thus, we can write:
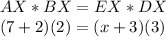
Now, we solve this for x:
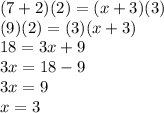
Thus,
DE = 3 units