If we evaluate the function
 at the given points, we have:
at the given points, we have:
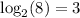

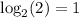
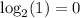

In fact, the function
 returns, for a given number
returns, for a given number
 , the exponent you have to give to 2 to obtain
, the exponent you have to give to 2 to obtain
 .
.
So, you want to obtain 8, you have to raise 2 to the 3rd power, if you want 4 you have to raise 2 to the 2nd power, and so on.