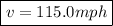Question

At NASA's Zero Gravity Research Facility in Cleveland, Ohio, experimental payloads fall freely from rest in an evacuated vertical

shaft through a distance of 132 m.
(a) If a particular payload has a mass of 50 kg, what is its potential energy relative to the bottom of the shaft?
(b) How fast will the payload be traveling when it reaches the bottom of the shaft?
m/s
(c) Convert your answer to mph for a comparison to highway speeds.
mph​

Answer 1

(a). Energy is 64,680 J

(b) velocity is 51.43m/s

(c) velocity in mph is 115.0mph

Step-by-step explanation:

(a).

The potential energy
`P` of the payload of mass
`m` is at a vertical distance
`h` is

`P =mgh`.

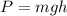Therefore, for the payload of mass
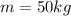`m = 50kg` at a vertical distance of
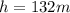`h = 132 m`, the potential energy is

`P = (50kg)(9.8m/s^2)(132m)`

`\boxed{P = 64,680J}`

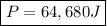
(b).

When the payload reaches the bottom of the shaft, all of its potential energy is converted into its kinetic energy; therefore,

`mgh= (1)/(2)mv^2`

`v= √(2gh)`

`v = √(2*9.8*135)`

`\boxed{v = 51.43m/s}`

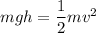
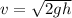
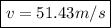
(c).

The velocity in mph is

`(51.43m)/(s) * (3600s)/(hr) * (1mile)/(1609.34m)`

`\boxed{v= 115.0mph}`