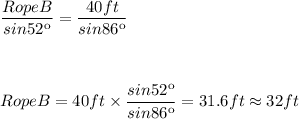Question

Two ropes are attached to a large ballon in a parade. The people holding the ropes are 40 feet apart,and the angle between the two ropes is 86 degrees. The angle of elevation of one rope is 42 degrees. How long is each rope..Step by Step explanation..Please ..Thanks.

Answer 1

The lenthts of the ropes, as per the figure attached, are

• 27 ft (rope A) and
• 32ft (rope B)

Step-by-step explanation:

See the figure attached for any reference.

We can find the lengths of both ropes using the law of sines.

1. Rope A:

`(RopeA)/(sin42\º)=(40ft)/(sin86\º)\\ \\\\\\RopeA=40ft * (sin42\º)/(sin86\º)=26.8ft\approx 27ft`

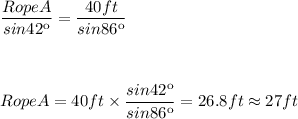
2. Rope B

First, calculate the included angle: 180º - 86º - 42º = 52º

Now use the law of sines:

`(RopeB)/(sin52\º)=(40ft)/(sin86\º)\\ \\\\\\RopeB=40ft * (sin52\º)/(sin86\º)=31.6ft\approx 32ft`