Question

What is the degree of the polynomial?

5x^4-3x^2+2x+1

A: 1
B: 2
C: 4
D:3

Answer 1

The degree of the polynomial
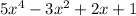`5x^4-3x^2+2x+1` is C. 4.

Step-by-step explanation:

A polynomial is a mathematical expression consisting of variables, coefficients, and exponents, combined using addition, subtraction, multiplication, and non-negative integer exponents. The degree of a polynomial is the highest power of its variable with a non-zero coefficient. It is determined by examining the exponent of the term with the greatest power. The degree provides insights into the complexity and behavior of the polynomial.

The degree of a polynomial is the highest power of the variable in the polynomial.

In this case, the highest power of x is 4, so the degree of the polynomial
`5x^4-3x^2+2x+1` is 4.

Answer 2

C: 4

Step-by-step explanation:

To find the degree of the polynomial, put the exponents in order from largest to smallest

5x^4-3x^2+2x+1

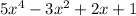The degree is the largest power, or in this case, the largest power is 4, so the degree is 4