Question

Ahhhh someone please help me! I was just getting the hang of it when it said that my answer is wrong! I don't understand what I did wrong please help me.

I multiplied all the y-axis by -1.

What am I missing?

What are the correct coordinates?


Please show all the work on how you got your answer.

Answer 1

Explanation:

The green triangle is JKL, right? You have plotted it correctly.

Now, for the reflection about x = -1:

First, imagine or draw the line x = -1. It is a line parallel to the y - axis and passing through the point (x,y) = (-1,0)

As the reflection is about a line parallel to the y - axis, the y co-ordinates of the original and reflected figures will be the same. Only the x co-ordinates of the reflected figure would change from the original one.

The formula to compute the x co-ordinate of the reflected geometry is:

`x_(reflected) = -1 - |x_(original) - (-1)| = -1 - |x_(original) + 1|`

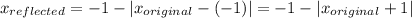
Applying this formula to J(2,-1):

`x_(reflected) = -1 - |2+1| = -1 -3 = -4`

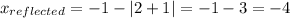
∴J' ≡ (-4,-1)

Similarly,

K' ≡ (-6,-5)

L' ≡ (-5,1)