Question

In order to estimate the average time spent on the computer terminals per student at a local university, data were collected from a sample of 64 business students over a one-week period. Assume the population standard deviation is 1.2 hours.a) With a 0.98 probability, the margin of error is approximately?b) If the sample mean is 10 hours, then the 98% confidence interval is approximately
?c) The standard error of the mean is?

Answer 1

a) 0.3495

b) (9.65, 10.35)

Explanation:

We are given the following in the question:

Sample mean,
`\bar{x}` = 10 hours

Sample size, n = 64

Alpha, α = 0.02

Population standard deviation, σ = 1.2 hours

a) Margin of error

Formula

`z_(critical)(\sigma)/(√(n))`

`z_(critical)\text{ at}~\alpha_(0.02) = \pm 2.33`

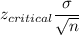
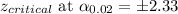
Putting values, we get,

`M.E = (2.33)(1.2)/(√(64)) = 0.3495`

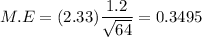
b) the sample mean is 10 hours, then the 98% confidence interval

`\mu \pm z_(critical)(\sigma)/(√(n))`

`\bar{x} \pm M.E`

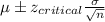
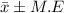
Putting the values, we get,

`10\pm 0.3495\\=(9.6505, 10.3495)\\\approx (9.65, 10.35)`