Answer:
D) Increase the sample size and decrease the confidence level
Explanation:
To build the confidence interval, initially we have to find the critical value of Z.
90% confidence interval
We have that to find our
 level, that is the subtraction of 1 by the confidence interval divided by 2. So:
level, that is the subtraction of 1 by the confidence interval divided by 2. So:

Now, we have to find z in the Ztable as such z has a pvalue of
 .
.
So it is z with a pvalue of
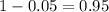 , so
, so
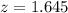
95% confidence interval
We have that to find our
 level, that is the subtraction of 1 by the confidence interval divided by 2. So:
level, that is the subtraction of 1 by the confidence interval divided by 2. So:

Now, we have to find z in the Ztable as such z has a pvalue of
 .
.
So it is z with a pvalue of
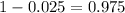 , so
, so
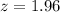
The width is
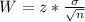
In which
 is the standard deviation of the population and n is the size of the sample.
is the standard deviation of the population and n is the size of the sample.
So, as z increses, so does the width. If z decreases, the width decreases. Lower confidence levels have lower values of z.
As n increases, the width decreses.
So the correct answer is:
D) Increase the sample size and decrease the confidence level