Question

A group of college DJs surveyed students to find out what music to plan for their upcoming parties. Thirty percent of the students preferred dubstep, 25% of the students liked trance music, and 20% wanted to hear only house music. Fifteen percent of the respondents selected both dubstep and trance.

A - The proportion of students that like trance music but not dubstep is:

a) 20% .
b) 5% .
c) 10% .
d) 15%B - The proportion of students that like either dubstep or trance is:

a) 40% .
b) 100% .
c) 55% .
d) 70% .

C - The proportion of students that like neither trance music nor dubstep is:

a)60% .
b) 20% .
c) 0% .
d) 40% .

D - The proportion of students that didn’t select any of the music options available on the survey was:
a) 0% .
b) 40% .
c) 20% .
d) 60% .

E - The proportion of students that preferred either dubstep or trance is calculated by:

a) P(A)+P(B)+P(A and B) .
b) None of the answer options are correct.
c) P(A)+P(B) .
d) P(A)+P(B)?P(A and B) .

F - The conditional probability that a student likes dubstep, given that he or she likes trance music, is:

a) 70% .

b) 65% .

c) 60% .

d) 55% .

Answer 1

Correct options are:

(A) (c)

(B) (a)

(C) (a)

(D) (d)

(E) (d)

(F) (c)

Explanation:

Denote the choice of music as follows:

D = a student likes dub-step

T = a student likes trance

H = a student likes house music.

The information provided is:

P (D) = 0.30

P (T) = 0.25

P (H) = 0.20

P (D ∩ T) = 0.15

(A)

Compute the probability of students that like trance music but not dub-step as follows:

`P(D^(c)\cap T)=P(T)-P(D\cap T)\\=0.25-0.15\\=0.10`

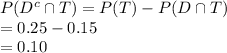
Thus, the probability of students that like trance music but not dub-step is 0.10.

(B)

Compute the probability of students that like either dub-step or trance as follows:

`P(D\cup T)=P(D)+P(T)-P(D\cap T)\\=0.30+0.25-0.15\\=0.40`

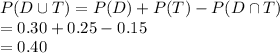
Thus, the probability of students that like either dub-step or trance is 0.40.

(C)

Compute the probability of students that like neither dub-step nor trance as follows:

`P(D^(c)\cup T^(c))=1-P(D\cup T)\\=1-0.40\\=0.60`

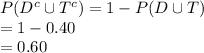
Thus, the probability of students that like neither dub-step nor trance is 0.60.

(D)

The probability of student selecting dub-step or trance is 0.40.

The probability of student selecting house music is 0.20.

Compute the probability that a student did not select (NS) any of the music options as follows:

P (D ∪ T) + P (H) + P (NS) = 1

0.40 + 0.20 + P (NS) = 1

P (NS) = 1 - 0.60

P (NS) = 0.40

Thus, the probability of students that didn’t select any of the music options available on the survey was 0.60.

(E)

The formula to compute the probability of students that like either dub-step or trance is:

P (D ∪ T) = P (D) + P (T) - P (D ∩ T)

The correct option is (d).

(F)

Compute the conditional probability that a student likes dub-step given that he/she likes trance as follows:

`P(D|T)=(P(D\cap T))/(P(T))=(0.15)/(0.25)=0.60`

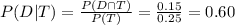
Thus, the conditional probability that a student likes dub-step given that he/she likes trance is 0.60.