Question

A thin uniform cylindrical turntable of radius 2.7 m and mass 22 kg rotates in a horizontal plane with an initial angular speed of 12 rad/s. The turntable bearing is frictionless. A clump of clay of mass 11 kg is dropped onto the turntable and sticks at a point 1.7 m from the point of rotation. Find the angular speed of the clay and turntable. Answer in units of rad/s. 010 (part 2 of 2) 10.0

Answer 1

8.59 rad/s

Step-by-step explanation:

Given that:

A thin uniform cylindrical turntable radius = 2.7 m

with a mass (M) = 22 kg

initial angular speed (ω₁) = 12 rad/s

Mass of the clump of the clay (m) = 11 kg

Diameter (d) from the point of rotation = 1.7 m

We are to find the final angular velocity (ω₂) ,To do that; we apply the conservation of annular momentum; which is as follows:

L₁ = L₂

l₁ω₁ = l₂ω₂

( 0.5 × M × r²) × ω₁ = (0.5 × M × r² + md²) ω₂

Making ω₂ the subject of the formula ; we have:

`\omega_2 = ((0.5*M*r^2)* \omega_1)/((0.5*M*r^2+md^2))`

`\omega_2 = ((0.5*22*2.7^2)* 12)/((0.5*22*2.7^2+11*1.7^2))`

`\omega_2 = 8.58 rad/s`

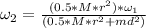
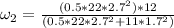
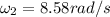
Hence, the angular speed of the clay and turntable = 8.59 rad/s