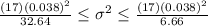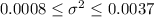Question

An automatic filling machine is used to fill bottles with liquid detergent. A random sample of 18 bottles results in a sample standard deviation of fill volume LaTeX: s\:=s =0.038 oz. Assume that fill volume is approximately normal. Determine the upper bound of a two-sided 97.5% confidence interval on the population variance, LaTeX: \sigma^2σ 2. Round your answer to four decimal places.

Answer 1

`((17)(0.038)^2)/(32.64) \leq \sigma^2 \leq ((17)(0.038)^2)/(6.66)`

`0.0008 \leq \sigma^2 \leq 0.0037`

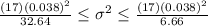
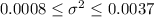
Explanation:

Data given and notation

s=0.038 represent the sample standard deviation

`\bar x` represent the sample mean

n=18 the sample size

Confidence=97.5% or 0.975

A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population mean or variance lies between an upper and lower interval".

The margin of error is the range of values below and above the sample statistic in a confidence interval.

The Chi Square distribution is the distribution of the sum of squared standard normal deviates .

Calculating the confidence interval

The confidence interval for the population variance is given by the following formula:

`((n-1)s^2)/(\chi^2_(\alpha/2)) \leq \sigma^2 \leq ((n-1)s^2)/(\chi^2_(1-\alpha/2))`

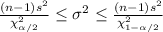
The next step would be calculate the critical values. First we need to calculate the degrees of freedom given by:

`df=n-1=18-1=17`

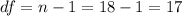
Since the Confidence is 0.975 or 97.5%, the value of
`\alpha=0.025` and
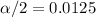`\alpha/2 =0.0125`, and we can use excel, a calculator or a table to find the critical values.

The excel commands would be: "=CHISQ.INV(0.0125,17)" "=CHISQ.INV(0.9875,17)". so for this case the critical values are:

`\chi^2_(\alpha/2)=32.64`

`\chi^2_(1- \alpha/2)=6.66`

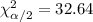
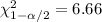
And replacing into the formula for the interval we got:

`((17)(0.038)^2)/(32.64) \leq \sigma^2 \leq ((17)(0.038)^2)/(6.66)`

`0.0008 \leq \sigma^2 \leq 0.0037`